1) Each mole of molecules contains N number of molecules, where N equals Avogadro's Number. How many molecules are in each answer: (a) 1 x N = N (b) 2 x N = 2N (c) 4 x N = 4N (d) N x 5 = 5N. 2) Each N times the number of hydrogen atoms in a formula equals the total number of hydrogen atoms in the sample: (a) N x 12 = 12N (b) 2N x 8 = 16N. We have to make a clear distinction between Avogadro's number and Avogadro's constant. The former is a unitless real number, defined as 6.02214076 × 10 23. The latter is a physical (or shall I call chemical?) constant, and thus has a unit. Also, how does an Avogadro’s number work into how stoichiometry is applied? Expert Answer SOLUTION Stoichiometry is derived from thelaw of conservation of masswhere the total mass of the reactants equals the total mass of the products.It is very important to understand the relation view the full answer.
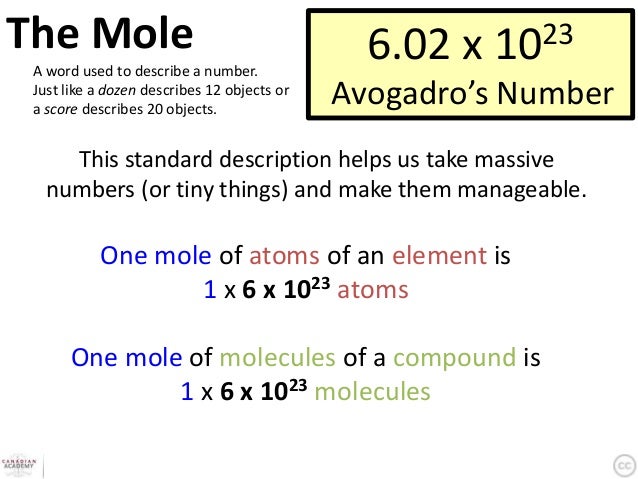
. This number is called Avogadro’s number. Thus, 1 mole of carbon atoms = 6.0221421 × 10 23 carbon atoms. Experimentally, 1 mole of 12 C has a mass of 12 g.
- Define stoichiometry.
- Identify the indicator information associated with stoichiometric molar relationships.
The first ten sections of this chapter presented and discussed three quantitative relationships involving the mole, which is a unit that relates a variety of chemically-significant numerical values and concepts to one another. Avogadro's number, which has a value of 6.02 × 1023, corresponds to the number of individual atoms, ions, or molecules that are present within a substance. 'Component within' molar relationships indicate the relative ratios of the elements that are present within a compound or molecule. Finally, atomic weight and molecular weight are molar quantities that relate to the mass of an element or a compound, respectively.
The final fundamental chemical quantity that will be discussed in this chapter is introduced below, and its associated equality pattern will be presented and applied in the following section.
Stoichiometry
The previous four sections of this chapter described and applied the process of balancing chemical equations. While the formulas of elements and compounds must change during a reaction, by definition, the relative quantity of each atom or ion involved in the reaction must be constant. The subscripts that are present within a chemical formula are solely dependent on the elemental, ionic, or covalent nature of the corresponding substance and, therefore, cannot be altered to uphold the Law of Conservation of Matter, which mandates that no particles be created or destroyed in the course of a chemical reaction. As a result, most reactions must be balanced, in order to account for any relative differences between the formulas of the reactants and products that are involved in the reaction.
Additionally, balancing coefficients indicate the ratio in which reactants must be present, in order to ensure a complete reaction, and dictate the number of product atoms or molecules that will be generated, relative to the amounts of reactants that are consumed. Stoichiometry, which is the quantitative study of substances as they participate in chemical reactions, is a fundamental chemical concept. Therefore, the balancing coefficients that are present in a reaction are chemically-significant quantities, and their corresponding chemical ratios are defined as molar standards.
Stoichiometry Indicator Information
Stoichiometry studies the relative molar ratios of the atoms and molecules that participate in chemical reactions. Unlike Avogadro's number, atomic weight,and molecular weight, which are indicated for use in a problem-solving context by the presence of specific key words, the indicator for stoichiometric molar relationships is highly generic: In order to apply a stoichiometric equality, the given problem, which must be associated with a chemical reaction, must refer to two chemicals that participate in that reaction.
For example, consider the following chemical equation.
Stoichiometry Avogadro's Number
(ce{2 H_2O_2} left( aq right) rightarrow ce{2 H_2O} left( l right) + ce{O_2} left( g right))
How many moles of O2 are produced from the decomposition of 7.53 moles of H2O2?
Because both of the chemicals that are referenced in the question, O2 and H2O2, are also present in the given reaction equation, a stoichiometric equality should be developed and applied when solving the problem.
Moles and Avogadro’s number are big! And they come across as overwhelming. But YOU can master them!
Avogadro's Number Worksheet
This video covers the definition of Moles, with an illustration showing the logic of moles in general chemistry. You’ll also see how to easily and quickly convert from and to them on the MCAT.
You’ll see conversions including atoms, AMU, grams, and moles. Let’s knock this out on your MCAT Content Category List!
(Watch on YouTube: Mole and Avogadro’s #. Click CC for transcription.)
How To Do Stoichiometry Step By Step
Links & Resources Mentioned In This Video:
Avogadro's Number Calculator
<– Watch Previous Video: Percent Mass by Composition
–> Watch Next Video: Density
Stoichiometry Using Avogadro's Number
This is Video 6 in the Stoichiometry & Reactions Video Series. Click HERE for the entire series.
Stoichiometry Using Avogadro's Number
Ready to test your knowledge? Try the MCAT Reactions and Stoichiometry practice quiz!
